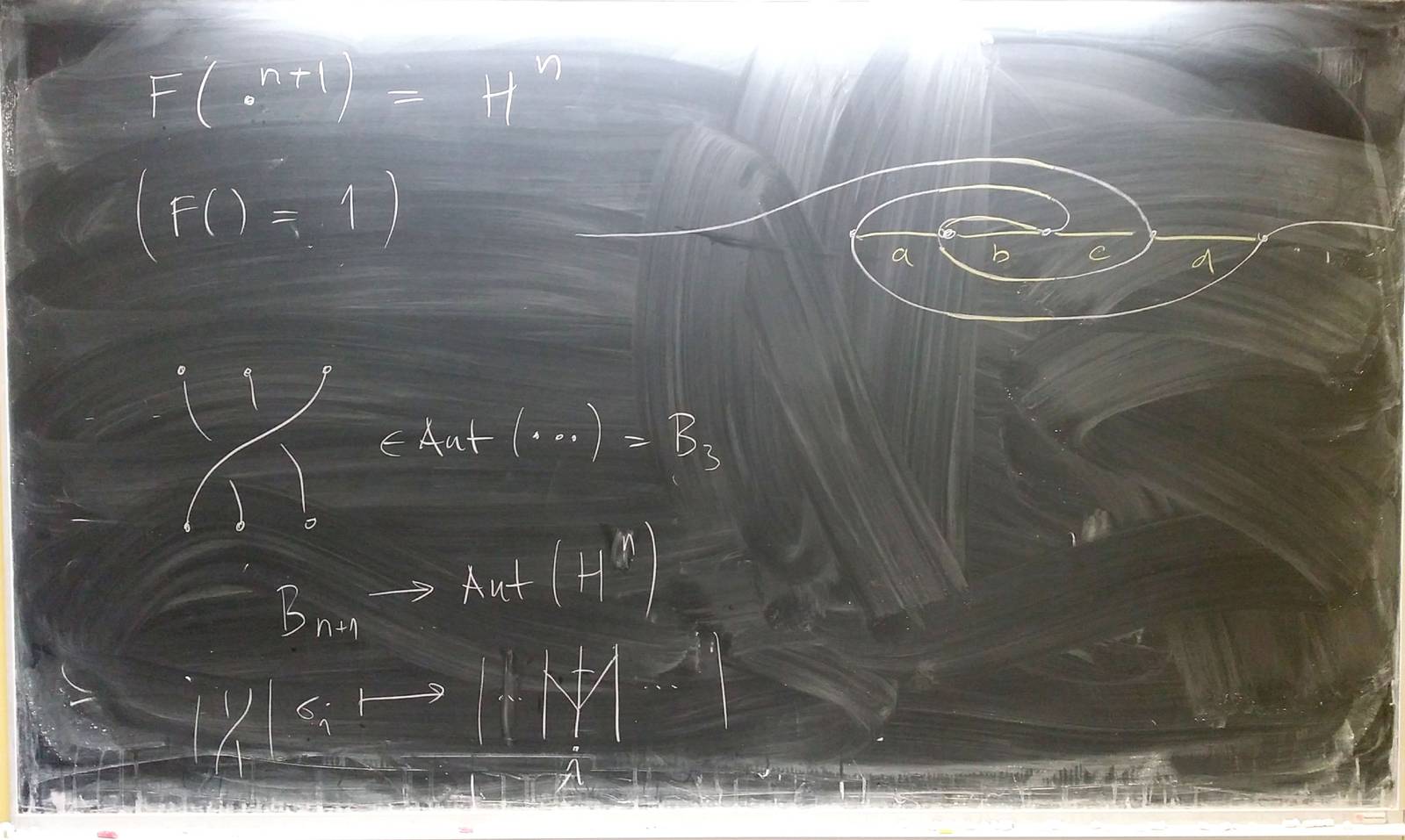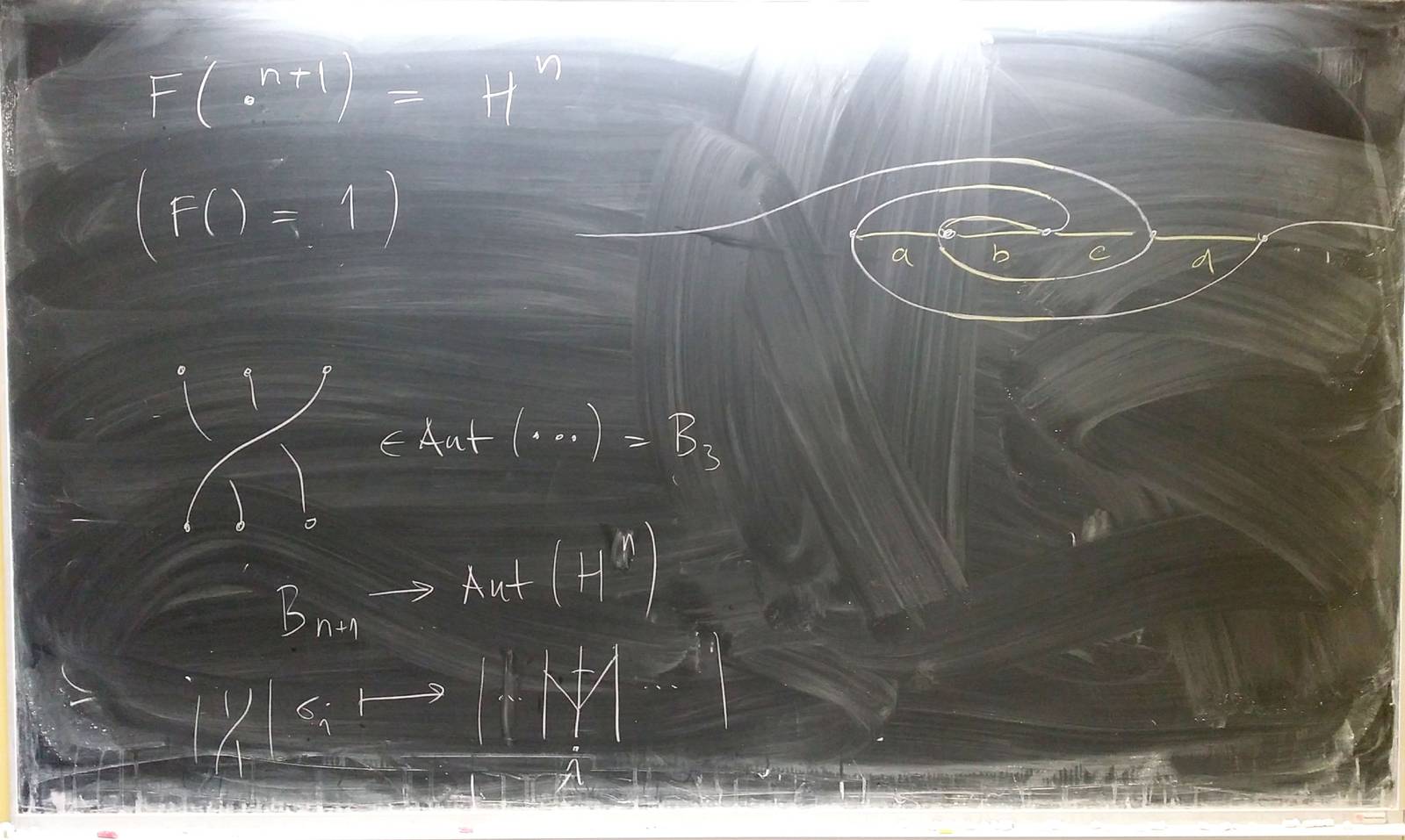
| Severa-{ | hide text |
| 131205-104541: | Getting the twist. |
| 131205-100511: | Explicit quantization formulas. |
| 131204-045438: | Quantizing Lie bialgebras - the universal construction (4). |
| 131204-045437: | Quantizing Lie bialgebras - the universal construction (3). |
| 131204-045436: | Quantizing Lie bialgebras - the universal construction (2). |
| 131204-045435: | Quantizing Lie bialgebras - the universal construction. |
| 131128-024041: | Quantizing Lie bialgebras (2). |
| 131125-065032: | Quantizing Lie bialgebras. |
| 131001-080619: | Quantizing quasi-Poisson structures (2). |
| 130927-105612: | Quantizing quasi-Poisson structures. |
| 130924-105132: | Some group level interpretations of associators. |
| 130924-102114: | A groupoid interpretation of $FL\times CW$. |
| 110202-112837: | The $A$ in Etingof-Kazhdan. |
| 110131-184732: | The Kontsevich vanishing lemma (3). |
| 110131-174434: | The Kontsevich vanishing lemma (2). |
| 110131-172714: | The Kontsevich vanishing lemma. |
| 110128-110538: | $\Phi_{AT}\in{\mathcal A}^{pb}$, day 2 (9). |
| 110128-104057: | $\Phi_{AT}\in{\mathcal A}^{pb}$, day 2 (8). |
| 110128-103444: | $\Phi_{AT}\in{\mathcal A}^{pb}$, day 2 (7). |
| 110128-102106: | $\Phi_{AT}\in{\mathcal A}^{pb}$, day 2 (6). |
| 110128-100631: | $\Phi_{AT}\in{\mathcal A}^{pb}$, day 2 (5). |
| 110128-095607: | $\Phi_{AT}\in{\mathcal A}^{pb}$, day 2 (4). |
| 110128-094323: | $\Phi_{AT}\in{\mathcal A}^{pb}$, day 2 (3). |
| 110128-093224: | $\Phi_{AT}\in{\mathcal A}^{pb}$, day 2 (2). |
| 110128-092351: | $\Phi_{AT}\in{\mathcal A}^{pb}$, day 2. |
| 110126-110302: | $\Phi_{AT}\in{\mathcal A}^{pb}$ (8) |
| 110126-105407: | $\Phi_{AT}\in{\mathcal A}^{pb}$ (7) |
| 110126-104058: | $\Phi_{AT}\in{\mathcal A}^{pb}$ (6) |
| 110126-102240: | $\Phi_{AT}\in{\mathcal A}^{pb}$ (5) |
| 110126-100927: | $\Phi_{AT}\in{\mathcal A}^{pb}$ (4) |
| 110126-095855: | $\Phi_{AT}\in{\mathcal A}^{pb}$ (3) |
| 110126-095118: | $\Phi_{AT}\in{\mathcal A}^{pb}$ (2) |
| 110126-093919: | $\Phi_{AT}\in{\mathcal A}^{pb}$ |
| } |