| Fiedler-{ | hide text |
| 221213-100013: | Is $V(K\setminus c)$ finite type? (4) |
| 221213-094244: | Is $V(K\setminus c)$ finite type? (3) |
| 221213-093927: | Is $V(K\setminus c)$ finite type? (2) |
| 221213-093920: | Is $V(K\setminus c)$ finite type? |
| 221129-132630: | Polynomial invariants which can distinguish the orientations of knots (7). |
| 221129-132625: | Polynomial invariants which can distinguish the orientations of knots (6). |
| 221129-132620: | Polynomial invariants which can distinguish the orientations of knots (5). |
| 221129-132615: | Polynomial invariants which can distinguish the orientations of knots (4). |
| 221129-132609: | Polynomial invariants which can distinguish the orientations of knots (3). |
| 221129-132605: | Polynomial invariants which can distinguish the orientations of knots (2). |
| 221129-132600: | Polynomial invariants which can distinguish the orientations of knots. |
| 170512-145638: | The latest 1-cocycle invariant (25). |
| 170512-145637: | The latest 1-cocycle invariant (24). |
| 170512-145636: | The latest 1-cocycle invariant (23). |
| 170512-145635: | The latest 1-cocycle invariant (22). |
| 170512-145634: | The latest 1-cocycle invariant (21). |
| 170512-145633: | The latest 1-cocycle invariant (20). |
| 170512-145632: | The latest 1-cocycle invariant (19). |
| 170512-145631: | The latest 1-cocycle invariant (18). |
| 170512-145630: | The latest 1-cocycle invariant (17). |
| 170512-145629: | The latest 1-cocycle invariant (16). |
| 170512-145628: | The latest 1-cocycle invariant (15). |
| 170512-145627: | The latest 1-cocycle invariant (14). |
| 170512-145626: | The latest 1-cocycle invariant (13). |
| 170512-145625: | The latest 1-cocycle invariant (12). |
| 170512-145624: | The latest 1-cocycle invariant (11). |
| 170512-145623: | The latest 1-cocycle invariant (10). |
| 170512-145622: | The latest 1-cocycle invariant (9). |
| 170512-145621: | The latest 1-cocycle invariant (8). |
| 170512-145620: | The latest 1-cocycle invariant (7). |
| 170512-145619: | The latest 1-cocycle invariant (6). |
| 170512-145618: | The latest 1-cocycle invariant (5). |
| 170512-145617: | The latest 1-cocycle invariant (4). |
| 170512-145616: | The latest 1-cocycle invariant (3). |
| 170512-145615: | The latest 1-cocycle invariant (2). |
| 170512-145614: | The latest 1-cocycle invariant. |
| 141002-094841: | Skype meeting along with Ester. |
| 140825-170444: | Last shot of Aug 2014. |
| 140825-105150: | A boundary? |
| 140825-100933: | Operations on finite type invariants. |
| 140825-094748: | Orienting R3 moves. |
| 140823-115508: | Invariants of connected sums. |
| 140823-103108: | A degree 1 cancellation. |
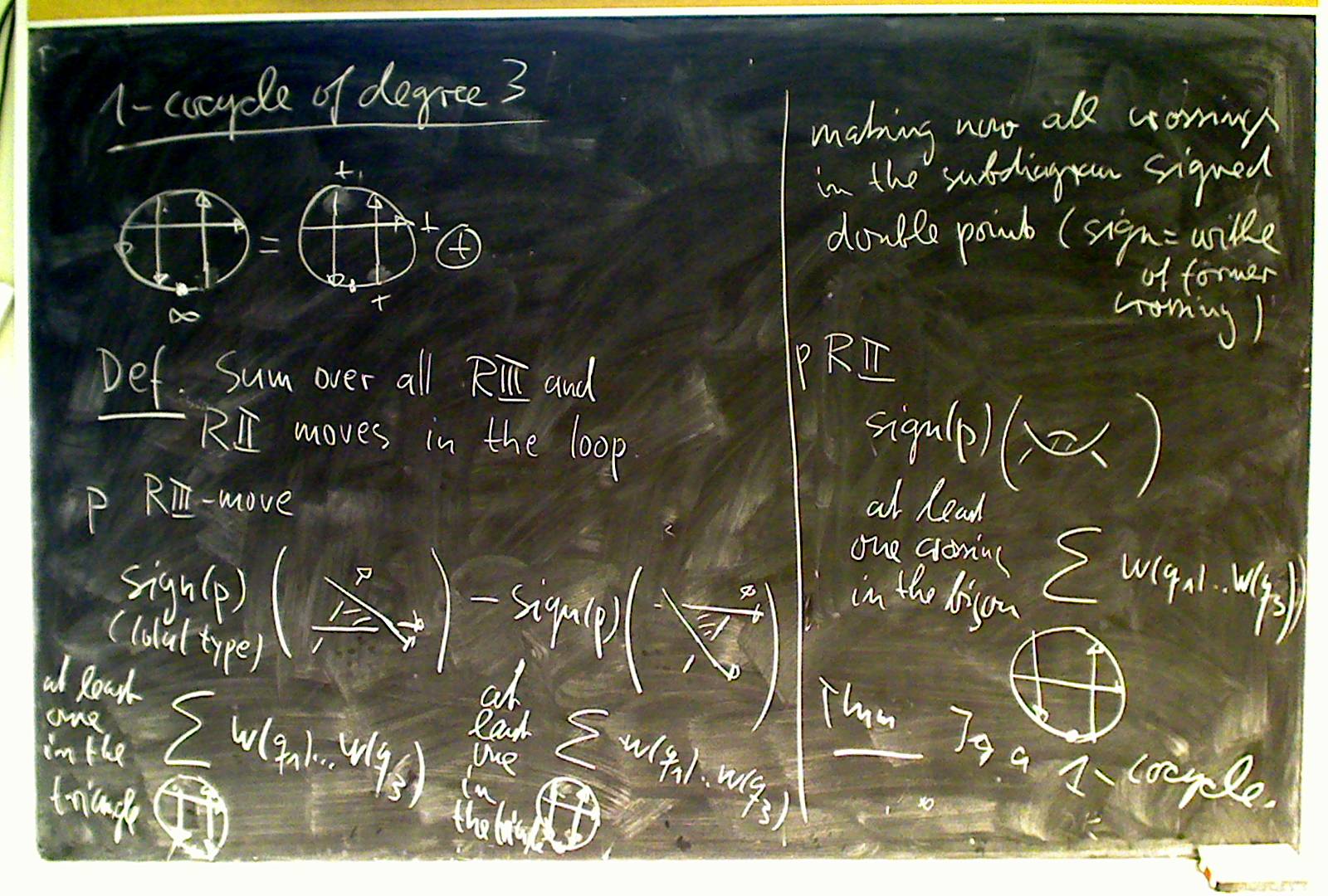
| 140823-101501: | A 1-cocycle of degree 3 (3). |
| 140823-094854: | A 1-cocycle of degree 3 (2). |
| 140823-093020: | A 1-cocycle of degree 3. |
| 140822-160404: | Bracelets. |
| 140822-101619: | The Fiedler-Whitney trick. |
| 140821-151908: | Dividing by weight systems??? |
| 140821-145927: | Dividing by weight systems, multi-local variations. |
| 140820-165548: | Braids (13). |
| 140820-164702: | Braids (12). |
| 140820-163626: | Braids (11). |
| 140820-162341: | Braids (10). |
| 140820-161703: | Braids (9). |
| 140820-160934: | Braids (8). |
| 140820-140714: | Braids (7). |
| 140820-135638: | Braids (6). |
| 140820-135409: | Braids (5). |
| 140820-134906: | Braids (4). |
| 140820-134334: | Braids (3). |
| 140820-133411: | Braids (2). |
| 140820-132545: | Braids. |
| 140820-130956: | $W_1$ and $W_2$. |
| 140820-113049: | The Tetrahedron Equation (2). |
| 140820-111723: | The Tetrahedron Equation. |
| 140820-105138: | Iteration and $v_2$ and $v_3$. |
| 140820-101653: | Kauffman-Vogel invariants. |
| 140820-100353: | A calculus for co-cycles (3). |
| 140820-095343: | A calculus for co-cycles (2). |
| 140820-094704: | A calculus for co-cycles. |
| 140819-154357: | Knot at Lunch: Singularization of Knots and Closed Braids (17). |
| 140819-154356: | Knot at Lunch: Singularization of Knots and Closed Braids (16). |
| 140819-154355: | Knot at Lunch: Singularization of Knots and Closed Braids (15). |
| 140819-154354: | Knot at Lunch: Singularization of Knots and Closed Braids (14). |
| 140819-154353: | Knot at Lunch: Singularization of Knots and Closed Braids (13). |
| 140819-154352: | Knot at Lunch: Singularization of Knots and Closed Braids (12). |
| 140819-154351: | Knot at Lunch: Singularization of Knots and Closed Braids (11). |
| 140819-154350: | Knot at Lunch: Singularization of Knots and Closed Braids (10). |
| 140819-154349: | Knot at Lunch: Singularization of Knots and Closed Braids (9). |
| 140819-154348: | Knot at Lunch: Singularization of Knots and Closed Braids (8). |
| 140819-154347: | Knot at Lunch: Singularization of Knots and Closed Braids (7). |
| 140819-154346: | Knot at Lunch: Singularization of Knots and Closed Braids (6). |
| 140819-154345: | Knot at Lunch: Singularization of Knots and Closed Braids (5). |
| 140819-154344: | Knot at Lunch: Singularization of Knots and Closed Braids (4). |
| 140819-154343: | Knot at Lunch: Singularization of Knots and Closed Braids (3). |
| 140819-154342: | Knot at Lunch: Singularization of Knots and Closed Braids (2). |
| 140819-154341: | Knot at Lunch: Singularization of Knots and Closed Braids. |
| 140819-104938: | Plans (10). |
| 140819-102407: | Plans (9). |
| 140819-101739: | Plans (8). |
| 140819-101112: | Plans (7). |
| 140819-100540: | Plans (6). |
| 140819-095813: | Plans (5). |
| 140819-094528: | Plans (4). |
| 140819-093218: | Plans (3). |
| 140819-092248: | Plans (2). |
| 140819-091110: | Plans. |
| 080621-154412: | A new Z/2 grading. |
| 080620-115025: | An even more general possibility. |
| 080620-115017: | The Z/2 grading (4). |
| 080620-114956: | The Z/2 grading (3). |
| 080619-163955: | The Z/2 grading (2). |
| 080619-163944: | The Z/2 grading. |
| 080619-090218: | Indexing permutations. |
| 080618-120425: | Further program details (2). |
| 080618-120418: | Further program details. |
| 080618-084419: | Further 1-parameter philosophy (5). |
| 080618-083549: | Further 1-parameter philosophy (4). |
| 080618-083544: | Further 1-parameter philosophy (3). |
| 080618-082520: | Further 1-parameter philosophy (2). |
| 080618-082510: | Further 1-parameter philosophy. |
| 080617-114051: | Program details. |
| 080617-084332: | One parameter philosophy (2). |
| 080617-084325: | One parameter philosophy. |
| 080616-162241: | Definition of the Y invariant (6). |
| 080616-154121: | Definition of the Y invariant (5). |
| 080616-154057: | Definition of the Y invariant (4). |
| 080616-154034: | Definition of the Y invariant (3). |
| 080616-134140: | Definition of the Y invariant (2). |
| 080616-134133: | Definition of the Y invariant. |
| 080616-133033: | An attempted Heegaard-Floer (5). |
| 080616-113840: | An attempted Heegaard-Floer (4). |
| 080616-113407: | An attempted Heegaard-Floer (3). |
| 080616-113400: | An attempted Heegaard-Floer (2). |
| 080616-111942: | An attempted Heegaard-Floer. |
| 080616-111937: | A state sum for Alexander-Jones (8). |
| 080616-110713: | A state sum for Alexander-Jones (7). |
| 080616-110704: | A state sum for Alexander-Jones (6). |
| 080616-104613: | A state sum for Alexander-Jones (5). |
| 080616-104606: | A state sum for Alexander-Jones (4). |
| 080616-103328: | A state sum for Alexander-Jones (3). |
| 080616-103319: | A state sum for Alexander-Jones (2). |
| 080616-102224: | A state sum for Alexander-Jones. |
| 080616-102039: | Alexander Kauffman for Tangles (3). |
| 080616-100835: | Alexander Kauffman for Tangles (2). |
| 080616-100827: | Alexander Kauffman for Tangles. |
| 080616-093820: | The Kauffman state model (3). |
| 080616-093447: | The Kauffman state model (2). |
| 080616-093440: | The Kauffman state model. |
| 080616-092144: | Kawauchi's theorem. |
| 080616-092137: | Ordinary crossings in 1-parameter knot theory. |
| 080616-090642: | Cabling. |
| 080616-090634: | White's theorem. |
| 080616-085744: | Regular isotopy. |
| 080616-085735: | Waldhausen's theorem. |
| 080616-084339: | 1-parameter knot theory. |
| 080616-084329: | 0-parameter knot theory (2). |
| 080616-084319: | 0-parameter knot theory. |
| } |
